[ad_1]
The big picture: Aside from Saturn’s iconic rings, several other planets also possess rings. Scientists still don’t fully understand their properties or how they form, and a recent discovery seriously challenges what they thought they knew.
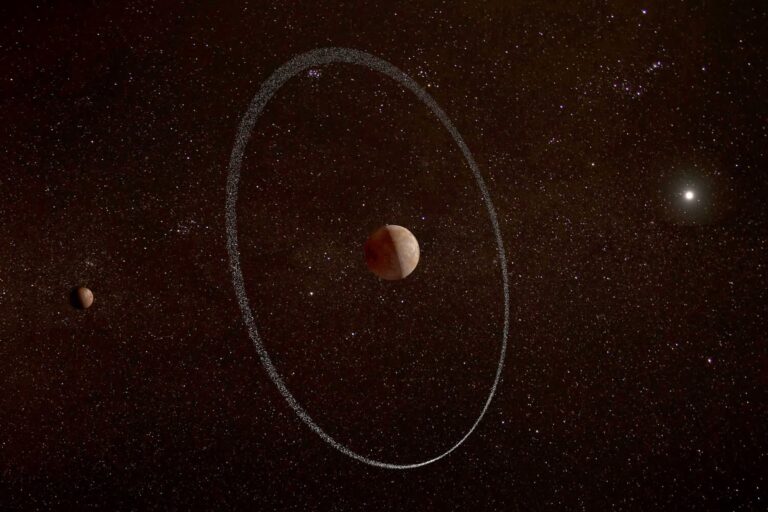
Dwarf planet Quaoar (pronounced “Kwawar”) lies far out beyond Neptune in a region of orbiting solar system objects known as the Kuiper Belt. With a diameter of around 690 miles (1,100km), Quaoar is the seventh-largest Kuiper Belt object – about half the size of nearby well-known Kuiper neighbor Pluto.
Astronomers recently confirmed the existence of a ring around Quaoar thanks to the European Space Agency’s (ESA) Characterising Exoplanet Satellite (Cheops) telescope. While watching Quaoar as it passed in front of distant stars, observers noticed that something else around the dwarf world also dimmed those stars. They verified that it was a ring by combining Cheops’s findings with data from ground-based telescopes taken between 2018 and 2021.
However, the discovery has astronomers baffled. Through tidal forces, celestial objects with strong enough gravity – like planets – will tear apart any smaller entity held together only by gravity that gets too close. We call the distance where that occurs the Roche Limit. Quaoar’s ring orbits at almost seven and a half times the planet’s radius, well beyond the distance science dictates moons to form.
Science has observed that rings tend to form inside the Roche Limit while moons orbit outside the boundary. We’ve seen this with other planets in our solar system, including Saturn and the dwarf planets Chariklo and Haumea. Quaoar’s ring lies beyond its Roche Limit, raising the question: Why hasn’t it coalesced into a second moon alongside the planet’s only known satellite, Weywot?
The ESA currently theorizes that the extreme cold at Quaoar’s orbiting distance – about 44 times the distance between Earth and the Sun – prevents the ice chunks comprising the ring from sticking together. The ESA admits more information is needed and that we should reconsider our understanding of ring systems and the Roche Limit.
[ad_2]
