Third-party presidential candidates are often blamed for ruining an election for one of the major political parties by “stealing” votes away from them. But with a little help from the math of social choice theory, elections could embrace the Ralph Naders of the world.

Take the historically tight Gore vs. Bush U.S. presidential election in 2000, when Americans anxiously awaited the resolution of legal battles and a recount that wouldn’t reveal a winner for another month. At the time the Onion published the headline “Recount Reveals Nader Defeated.” Of course Ralph Nader was never a serious contender for office. This led many to suggest that the Green Party candidate, like other third-party politicians, had attracted enough votes away from one of the two major parties to tip the scales against them (in this case the Democrats, who lost the election by just 537 votes).

Ranked choice voting is an alternative electoral system that would mitigate the spoiler effect while giving voters more voice to express themselves at the polls, social choice theorists suggest. Ranked choice voting boasts some obvious advantages. The mathematical discussion over how best to implement it, however, is surprisingly subtle.  And an old theorem from economics suggests that all attempts to use ranked choice voting are vulnerable to counterintuitive results.

Rather than voting only for a single candidate, ranked choice voting allows people to rank the candidates in order of preference. This way, if somebody wanted to support Nader but didn’t want to take away a vote from Gore, they could have ranked Gore second at the voting booth. When Nader didn’t amass enough votes, the second place ranking for Gore would still benefit him in the election. Proponents also contend that ranked choice voting disincentivizes vicious mudslinging campaign tactics. That’s because, unlike in typical elections, candidates in ranked choice races would need to appeal to all voters, even those who wouldn’t give them a top spot on the ballot. Currently Maine and Alaska have instituted ranked choice voting for all state and federal elections, including presidential primaries, in which it’s more likely for multiple candidates to appeal to the same voters.

Once voters have ranked candidates, how does that information get aggregated to reveal a single winner? The answer is not as straightforward as it might seem, and many schemes have been proposed. Let’s explore three approaches to vote tallying: plurality, instant runoff and “Condorcet methods,” each of which leads to unexpected behavior.

Unlike instant runoff and Condorcet methods, plurality is not actually a ranked choice voting scheme. In fact, it’s the most common voting method in the U.S.: whoever gains the most first-choice votes wins the election. Plurality can come with unfavorable outcomes. In addition to the problem of third-party election spoilers, imagine if candidate A received 34 percent of the votes, and candidates B and C each received 33 percent. Candidate A would win the plurality even if they were the last choice of every other voter. So 66 percent of the population would have their last pick for president. Plurality wastes votes and ignores the full spectrum of voter preferences. 

A better method (and the one used in American implementations of ranked choice voting as well as to select the best picture at the Oscars) is called instant runoff. If no candidate receives more than half of the first-choice votes, then the candidate with the fewest first-choice votes is removed from consideration and their votes get reallocated according to voters’ next choices (e.g., if your ranking of three candidates was 1) B, 2) C, 3) A, and candidate C gets removed for having the fewest first-choice votes, then A will get bumped up in your ballot to fill C’s gap: 1) B, 2) A). The process of removing the candidate with fewest first-choice votes repeats until one candidate has a majority. While instant runoff wastes fewer votes than plurality, it has drawbacks of its own. Bizarre situations can arise where your favorite candidate is more likely to lose if they get more first-choice votes. 

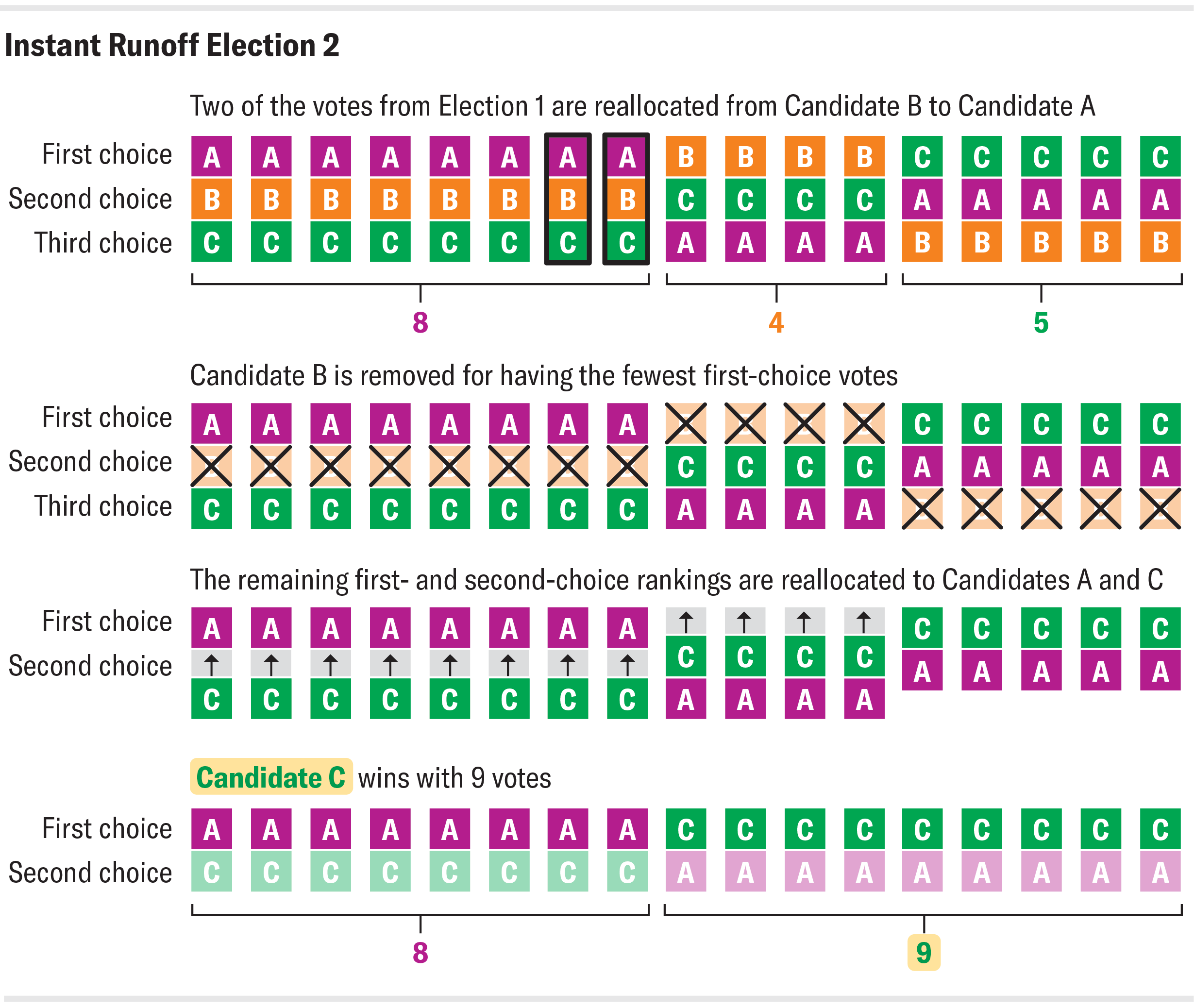
The figure below (based on an example from electionscience.org) depicts this scenario. In a hypothetical instant runoff election, called Election 1, candidate A wins. Although A and B have the same number of first-choice votes, neither has the majority required to win (nine out of 17 votes). So C, having the fewest first-choice votes is removed from contention, and the ballots are adjusted to fill C’s gaps, yielding a majority for candidate A.

Now imagine a separate scenario called Election 2, where everybody votes in the same way as in Election 1 except for two people who upgrade A from their third choice to their first choice. Amazingly, even though A won Election 1 and now has more first-choice votes than before, C becomes the new victor. This is because upgrading A results in downgrading B so that B is now the candidate with the fewest first-choice votes. When B is removed, A doesn’t fare as well in a head-to-head against C as they did against B in Election 1.

This counterintuitive phenomenon occurred in Burlington, Vt.’s 2009 mayoral election where Progressive Party member Bob Kiss beat the Republican and Democratic nominees in an instant runoff election. Amazingly, if more voters had placed Kiss first in their ranking, he would have lost the election.

Condorcet methods, named after 18th-century French mathematician and political philosopher Marquis de Condorcet, elect a candidate who would win in a head-to-head election against any other candidate. For example, suppose that in an election without any third-party candidates Al Gore would have beaten George W. Bush in 2000. Gore certainly would have won head-to-head contests with any one of the third-party candidates as well. Gore winning any hypothetical one-on-one election would make him a so-called Condorcet candidate. It seems obvious that such a candidate should be the victor because the population prefers them to all of their opponents. But here’s an important snag in electing Condorcet candidates: they don’t always exist. Voter preferences can be cyclic such that the population prefers A to B and B to C but also prefers C to A. This violation of transitivity is known as the Condorcet paradox (and is reminiscent of intransitive dice, which I wrote about recently).

Several other schemes for amalgamating ranked votes into a winner have been proposed. They each have their pros and cons, and, even more unsettling, the outcome of an election can entirely depend on which system gets used.

The figure shows a simple election yielding different results under each of the three systems we’ve discussed. This comparison leads to a hopeful question: Is there a perfect ranked-choice voting system? Perhaps we just have yet to discover the ideal design that maximally represents the interest of the public while avoiding the pitfalls of existing proposals. Nobel Prize–winning economist Kenneth Arrow investigated this question and came up with bare minimum requirements of any reasonable aggregator, or method that converts voter rankings into one societal ranking: 

1. Unanimity: If every person in the population ranks candidate A above candidate B, then the aggregator should put candidate A above candidate B. 
2. Independence of irrelevant alternatives: Suppose the aggregator puts candidate A above B. If voters were to change some of their rankings but everybody kept their relative order of A vs. B the same, then A should remain above B in the new aggregate ranking. The societal ordering of A and B should depend only on individual orderings of A and B and not those of other candidates. 

The commonsensical list sets a low bar. But Arrow proved a striking fact that has come to be known as Arrow’s impossibility theorem: there is only one type of aggregator that satisfies both conditions, a dictatorship. By a dictatorship, Arrow means a ridiculous aggregator that simply always mimics the rankings of the same single voter. No ranked choice voting scheme, regardless of how clever or complex it is, can simultaneously satisfy unanimity and independence of irrelevant alternatives unless it’s a dictatorship. The theorem suggests that no ranked choice voting scheme is perfect, and we will always have to contend with undesirable or counterintuitive outcomes. 

This proof doesn’t mean that we should scrap ranked choice voting. It still does a better job at capturing the will of the electorate than plurality voting. It simply means that we need to pick and choose which properties we want in our aggregator and acknowledge that we can’t have it all. The theorem doesn’t say that every single election will be flawed, but rather that no ranked-choice electoral system is invulnerable to flaws. Arrow has said of his own result: “Most systems are not going to work badly all of the time. All I proved is that all can work badly at times.”

And the idealists among us should not lose hope, because ranked choice voting isn’t the only game in town for souped-up electoral systems. Merely ranking candidates loses a lot of information about voter preferences. For example, here’s a ranking of foods I’d like to eat from most desirable to least: vanilla ice cream > chocolate ice cream > my own sneakers. The ranking conveys no information about just how close my taste for vanilla and chocolate ice cream is nor my distaste for my Nikes. To properly express my desires, I should assign scores to the candidate meals to communicate not only which ones I prefer more but by how much. This is called cardinal voting, or range voting, and although it’s no panacea and has its own shortcomings, it circumvents the limitations imposed by Arrow’s impossibility theorem, which only applies to ranked choice voting. 

We’re all familiar with cardinal voting. In Olympic gymnastics, judges decide the winner by giving numeric scores to the competitors, and whoever has the most total points at the end wins. Whenever you sort products by consumers’ star ratings online you’re presented with the winners of a cardinal election. Ancient Spartans elected leaders by gathering in an assembly and shouting for each of the candidates in turn. Whoever received the loudest shouts won the election. While this sounds crude to modern ears, it was actually an early form of cardinal voting. People could vote for multiple candidates and “score” them by choosing what volume of voice to contribute to the roar of the crowd. This is impressive considering that cardinal voting, despite granting the people more input than any of the other systems we’ve discussed, has never been used in a modern election. Perhaps we shouldn’t be surprised: the ancient Greeks did invent democracy after all.